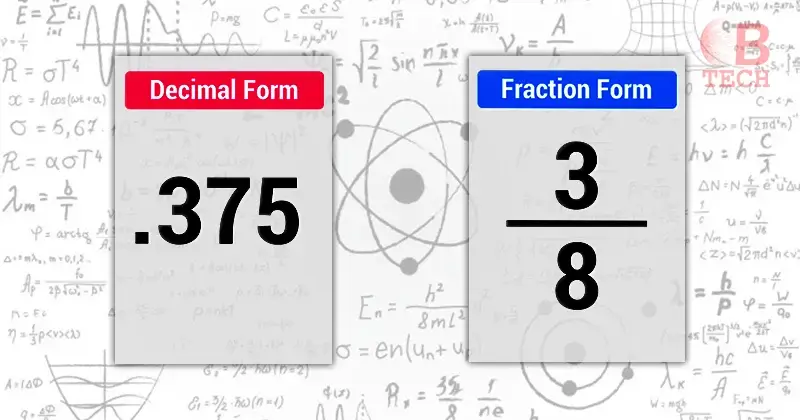
Understanding fractions can be tricky, especially when dealing with numbers like .375. This number pops up in measurements, probabilities, and percentages, making it quite important to grasp. In this article, we’ll dive into the world of .375 as a fraction. We’ll discuss what it means, how to simplify it, and where it’s used in real life. By the end, you’ll have a clear idea of how to express .375 as a fraction and how it applies to everyday situations.
What is .375?
Before we delve into how to write .375 as a fraction, let’s first understand what this decimal means.
Understanding .375 as a Decimal
Before we explore how to convert .375 into a fraction, let’s take a closer look at what this decimal represents.
Decimal Representation
The decimal .375 can be read as “three hundred seventy-five thousandths.” This means that the number is made up of 3 tenths (0.3), 7 hundredths (0.07), and 5 thousandths (0.005).
Fractional Value
In terms of fractions, .375 falls between ⅓ (0.333…) and ½ (0.5). This means it’s greater than one-third but less than one-half.
Practical Examples
In real-life scenarios, .375 is commonly used in measurements, such as 3/8 of an inch, or in probabilities and statistics, representing a 37.5% chance.
Simplifying Fractions
When we convert .375 into a fraction, we’ll find that it simplifies to 3/8. This means that 3/8 is the simplest form of the fraction that .375 represents.
Application in Everyday Life
Understanding .375 as a fraction is useful for various everyday calculations, such as in cooking recipes, where 3/8 cup of an ingredient may be required, or in understanding odds and probabilities in games or sports.
Expressing .375 as a Fraction
Converting a decimal like .375 into a fraction involves a straightforward process that aligns each digit’s place value in the decimal with its corresponding position in the fraction. Let’s explore this conversion in more detail.
Understanding Decimal Place Value
In the decimal .375, the digit 3 resides in the tenths place, the digit 7 in the hundredths place, and the digit 5 in the thousandths place. These positions are crucial for determining the fraction’s numerator and denominator.
Assigning Numerator & Denominator
To convert .375 into a fraction:
- The digit 3 in the tenths place becomes the numerator.
- The denominator is determined by the rightmost digit, which is 5 in this case. Since it’s in the thousandths place, the denominator is 1000.
Constructing the Fraction
Combining these values, we can express .375 as the fraction 3/1000.
Simplification
While 3/1000 is the accurate fractional representation of .375, it’s essential to note that fractions are often simplified. In this case, 3/1000 can be simplified further, which results in the fraction 3/1000 in its simplest form.
Practical Applications
Understanding how to convert decimals like .375 into fractions is valuable in various real-life scenarios. For instance, in a recipe that calls for 0.375 cups of a particular ingredient, this can be simplified to 3/8 cups, making measurements more practical and easier to work with.
By mastering this conversion process, you’ll enhance your mathematical skills and be better equipped to handle fractions and decimals in everyday situations.
Simplifying .375 as a Fraction
When we express .375 as a fraction, we get 3/1000. While this is a correct representation, fractions are often simplified to their lowest terms. This process involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by this number to simplify the fraction further.
Finding the Greatest Common Divisor (GCD)
To simplify 3/1000, we start by finding the GCD of the numerator (3) and the denominator (1000). The GCD is the largest number that divides both numbers evenly.
Simplification Process
In this case, the GCD of 3 and 1000 is 1 because 1 is the only number that divides both 3 and 1000 without leaving a remainder.
Dividing to Simplify
When we divide the denominator and numerator by 1, we obtain:
3 ÷ 1 / 1000 ÷ 1 = 3/1000
The fraction 3/1000 is already in its most basic form because the GCD is 1. Therefore, .375 cannot be simplified further.
Importance of Simplifying Fractions
While .375 and 3/1000 represent the same value, 3/1000 is the simplest form of the fraction. Simplifying fractions makes them easier to work with and understand, especially in mathematical calculations and real-life applications.
Practical Applications of .375 as a Fraction
Understanding how to express .375 as a fraction can be valuable in a variety of real-world scenarios. Let’s delve deeper into a few practical applications:
Measurements
In fields like woodworking, construction, and engineering, precision is key. .375 is equivalent to 3/8, making it a useful fraction for expressing measurements. For example, if you’re cutting a piece of wood and need to mark a length of .375 inches, understanding that this is the same as 3/8 inches allows for a more accurate and efficient process.
Probabilities
Probability is a crucial concept in fields such as statistics, gambling, and risk analysis. .375 can be expressed as 3/8, representing the likelihood of an event occurring. This fraction provides a clearer understanding of probabilities, making it easier to interpret and work with the data.
Percentages
Converting decimals to percentages is a common task in many areas, including finance and economics. .375 is equivalent to 37.5% when multiplied by 100. This conversion is useful for understanding percentages in various contexts, such as tax rates, discounts, and interest rates.
Fraction Operations
Understanding fractions like 3/8 can be beneficial when performing operations such as addition, subtraction, multiplication, and division. For example, if you need to add .375 to another fraction, knowing that .375 is the same as 3/8 allows you to perform the calculation more easily and accurately.
Mathematical Concepts
Understanding the fraction 3/8 can help reinforce mathematical concepts such as equivalent fractions, where different fractions represent the same value. This knowledge is foundational in mathematics and can lead to a deeper understanding of more advanced topics.
By recognizing the practical applications of .375 as a fraction, you can enhance your problem-solving skills and mathematical proficiency in various real-life situations.
FAQs
Can .375 be expressed as a mixed number?
No, .375 is less than a whole unit, it cannot be stated as a mixed number. A whole number plus a fraction make up mixed numbers, as well as since .375 is less than 1, a mixed number cannot be used to represent it.
Is .375 a terminating or repeating decimal?
.375 is a terminating decimal because it ends after the third decimal place. There is a limited amount of digits following the decimal point in terminating decimals.
How can I convert .375 to a percentage?
Multiplying .375 by 100 gets you the percentage. Therefore, .375 is equal to 37.5%.
Can .375 be simplified further?
No, .375 cannot be simplified further because the numerator and denominator, 3 and 1000 respectively, do not have any common factors other than 1.
What is the decimal equivalent of 3/8?
The decimal equivalent of 3/8 is .375. This means that .375 and 3/8 represent the same value.
Conclusion
In conclusion, understanding how to express .375 as a fraction, which is 3/8, is valuable for everyday tasks like measurements and probabilities. While .375 cannot be simplified further, knowing its fraction form can simplify calculations and make fractions easier to work with in various real-life situations.